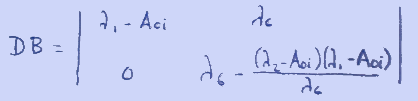Theory
Theory
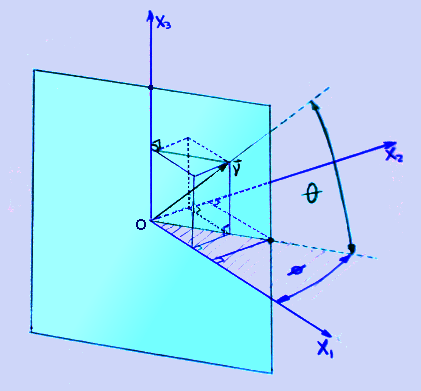
Let's consider a vertical plane defined by a phi rotation around the X3 axis.
The unit vector is defined:
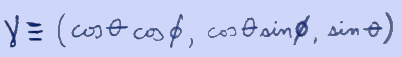
Let's consider also the following convention for the stiffness tensor and the symmetric condition:
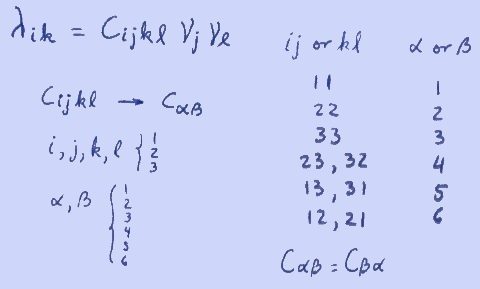
We can also use that convention for lambda. Defining l, m and n as follows and expanding for lambda 1 to 6 we have:
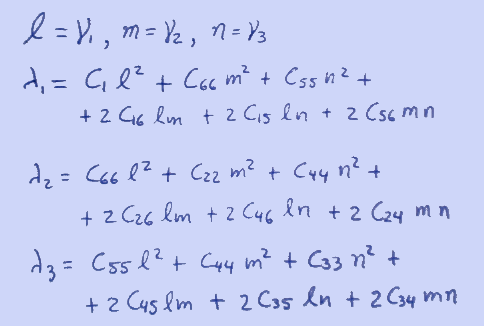
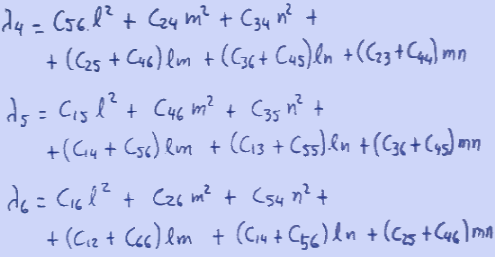
From the Christofell's equation we have:
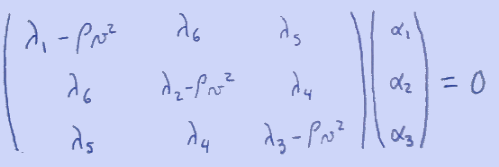
And the determinant must be zero:
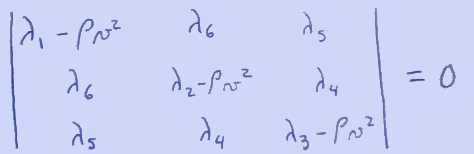
Defining A as follows, expanding the determinant we arrive to the following polynomial in A:

Now let's define the polynomial coefficients:
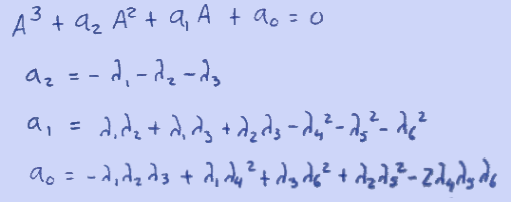
In order to find the roots, we will find first the location in A of the maximum and minimum values of the polynomial, by taking the derivative:

The three roots

of the original third grade polynomial will be located one at the left of A1, one in between of A1 and A2, and one at the right of A2.
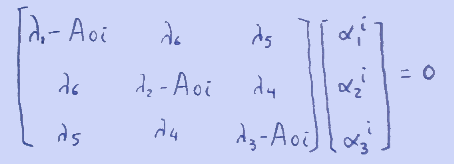
Now, we can express the following:
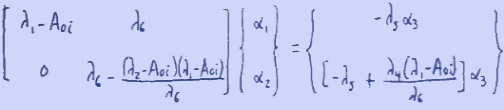
and considering that alfa is a unit vector:
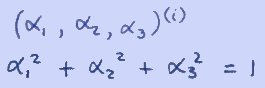
We arrive to the following results for alfa:
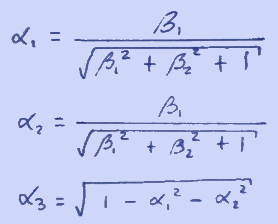
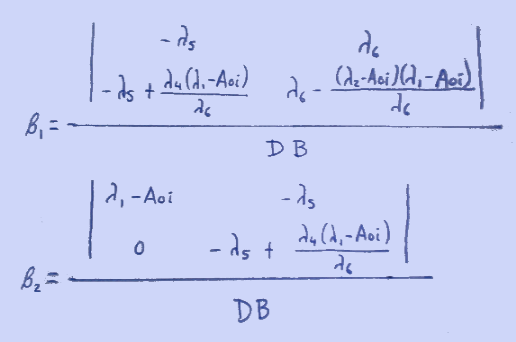
wn which beta1, beta2 and DB are defined: